How to work out area

One to one maths interventions built for KS4 success

Weekly online one to one GCSE maths revision lessons now available

Learn more

In order to access this I need to be confident with:

2D shapes Rounding Arithmetic Substituting numbers into formulae Converting between metric units BIDMAS

This topic is relevant for:

GCSE Maths Geometry and Measure Area

How To Work Out Area

Here we will learn how to work out area.

There are also how to work out area worksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to go next if you’re still stuck.

What is area?

Area is a measure of how much space there is inside of a $2$ dimensional shape. To find the area of a shape we can either count the number of unit squares within the shape or use the appropriate area formula for that shape.

Area is measured in square units e.g. $cm^{2}, \; m^{2}, \; mm^{2}$ .

The table below shows the formulae for calculating area for some of the most common $2$ D shapes:

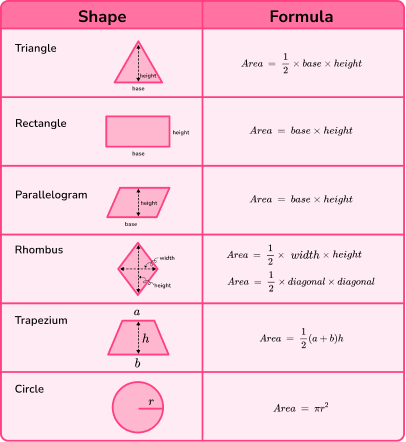
What is area?

How to work out area

In order to work out area:

Write down the formula.
Substitute the values into the formula.
Do the calculation.
Write the answer, including the units.

How to work out area

How to work out area worksheet

Get your free how to work out area worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

How to work out area worksheet

Get your free how to work out area worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Related lessons on area

How to work out area is part of our series of lessons to support revision on area. You may find it helpful to start with the main area lesson for a summary of what to expect, or use the step by step guides below for further detail on individual topics. Other lessons in this series include:

How to work out area examples

Example 1: area of a rectangle

Work out the area of the rectangle

Write down the formula.

\text{Area }= base \times height

2Substitute the values into the formula.

Here the base is $11cm$ and the height is $4cm.$

Area=11 \times 4

3Do the calculation.

Area=11 \times 4

Area=44

4Write the answer, including the units.

The measurements are in centimetres so the area will be measured in square centimetres.

Area=44cm^2

Example 2: area of a parallelogram

Work out the area of the parallelogram

Write down the formula.

Show step

Area= base \times height

Substitute the values into the formula.

Show step

Here the base is $10m$ and the height is $6m.$

$Area=10 \times 6$

Do the calculation.

Show step

Area=10 \times 6

$Area=60$

Write the answer, including the units.

Show step

The measurements are in metres so the area will be measured in square metres.

$Area=60m^2$

Example 3: area of a rhombus

Calculate the area of the rhombus

Write down the formula.

Show step

\text{Area }=\frac{1}{2} \times \text{ width } \times \text{ height}

or $\text{Area }= \frac{1}{2} \times \text{ diagonal } \times \text{ diagonal}$

Substitute the values into the formula.

Show step

Here the width is $20mm$ and the height is $8mm.$

$\text{Area }=\frac{1}{2} \times 20 \times 8$

Do the calculation.

Show step

\begin{aligned} \text{Area }&=\frac{1}{2} \times 20 \times 8\\\\ \text{Area }&=80 \end{aligned}

Write the answer, including the units.

Show step

The measurements are in millimetres so the area will be measured in square millimetres.

$Area=80mm^2$

Example 4: area of a trapezium

Find the area of the trapezium

Write down the formula.

Show step

\text{Area }=\frac{1}{2}(a+b)h

Substitute the values into the formula.

Show step

In a trapezium, $a$ and $b$ are the parallel sides and $h$ is the height.

Here $a=3, \; b=5$ and $h=4$

$\text{Area }=\frac{1}{2}(3+5) \times 4$

Do the calculation.

Show step

\begin{aligned} &\text{Area }=\frac{1}{2}(3+5) \times 4\\\\ &\text{Area }=\frac{1}{2} \times 8 \times 4\\\\ &\text{Area }=16 \end{aligned}

Write the answer, including the units.

Show step

The measurements are in $cm$ so the area will be measured in $cm^2$ .

$Area=16cm^2$

Example 5: area of a triangle

Work out the area of the triangle

Write down the formula.

Show step

\text{Area }=\frac{1}{2} \times \text{ base } \times \text{ height}

Substitute the values into the formula.

Show step

Since this is a right triangle, we can see the base of the triangle is $13mm$ and the height of the triangle is $6mm$ .

$\text{Area }=\frac{1}{2} \times 13 \times 6$

Do the calculation.

Show step

\begin{aligned} \text{Area }&=\frac{1}{2} \times 13 \times 6\\\\ \text{Area }&=39 \end{aligned}

Write the answer, including the units.

Show step

The measurements are in $mm$ so the area will be measured in $mm^2$ .

$Area=39mm^2$

Example 6: area of a circle

Work out the area of the circle. Give your answer to $1$ decimal place

Write down the formula.

Show step

\text{Area }=\pi r^{2}

Substitute the values into the formula.

Show step

Here the radius, $r,$ is $3.5cm$ .

$\text{Area }=\pi \times 3.5^{2}$

Do the calculation.

Show step

\begin{aligned} \text{Area }&=\pi \times 3.5^{2}\\\\ \text{Area }&=38.48451001 \end{aligned}

Write the answer, including the units.

Show step

We need to write the answer to one decimal place and include the units.

$Area=38.5cm^2$

How to work out area of a compound shape

In order to work out area of a compound shape:

Draw lines to split the shape into two or more smaller shapes. Label the shapes A, B, C, …
Consider each shape individually.
a) Work out any measurements that you need.
b) Calculate the area using the methods above.
Add or subtract the relevant areas to find the total area.
Write the answer, including the units.

Area of a compound shape examples

Example 7: area of a compound shape

Work out the area of the following shape

Draw lines to split the shape into two or more smaller shapes. Label the shapes A, B, C, …

Show step

Consider each shape individually.

Show step

Shape A

a) Work out any measurements that you need.

For a rectangle, we need the height and the base,both of which we are given.

b) Calculate the area using the methods above.

$\begin{aligned} \text{Area }&=\text{ base }\times \text{ height}\\\\ &=10 \times 6\\\\ &=60\mathrm{cm}^{2} \end{aligned}$

Shape B

a) Work out any measurements that you need.

For a trapezium, we need $a, \; b$ and $h.$

b) Calculate the area using the methods above.

$\begin{aligned} \text{Area }&=\frac{1}{2}(a+b)h\\\\ &=\frac{1}{2}(5+10) \times 3\\\\ &=22.5\mathrm{cm}^{2} \end{aligned}$

Add or subtract the relevant areas to find the total area.

Show step

Total area: $60+22.5=82.5$

Write the answer, including the units.

Show step

\text{Area } =82.5cm^2

Example 8: area of a compound shape

Work out the shaded area. Give your answer to one decimal place.

Draw lines to split the shape into two or more smaller shapes. Label the shapes A, B, C, …

Show step

The shape is already clearly split.

Consider each shape individually.

Show step

Shape A

a) Work out any measurements that you need.

For a triangle we need the height and base, which we are given

b) Calculate the area using the methods above.

$\begin{aligned} \text{Area }&= \frac{1}{2} \times \text{ base } \times \text{ height}\\\\ &=\frac{1}{2} \times 10 \times 14\\\\ &=70\mathrm{cm}^{2} \end{aligned}$

Shape B

a) Work out any measurements that you need.

Here we need the radius of the circle, which is $3.2cm$

b) Calculate the area using the methods above.

$\begin{aligned} \text{Area }&=\pi \times r^{2}\\\\ &=\pi \times 3.2^{2}\\\\ &=32.16990877\\\\ &=32.17\mathrm{cm}^{2} \end{aligned}$

Add or subtract the relevant areas to find the total area.

Show step

Shaded area: $70-32.17=37.83$

Write the answer, including the units.

Show step

\text{Area } =37.8cm^2

Common misconceptions

Using an incorrect formula

There are several different formulae for the different shapes – make sure you use the correct one.

Using the incorrect units/not including units

Area is measured in square units.

E.g.

Square millimetres, square centimetres, square metres, square inches, square feet, square yards etc.

Calculating with different units

All measurements must be in the same units before calculating surface area.

E.g.

You can’t have some measurements in $cm$ and some in $m.$

Calculating perimeter/circumference instead of area

Remember, perimeter is distance around the outside whilst area is the space inside the shape.

Using diameter instead of radius for a circle

To work out the area of a circle we need the radius, which is the distance from the centre of the circle to the edge of the circle.

Practice how to work out area questions

14\mathrm{cm}^{2}

49\mathrm{cm}^{2}

28\mathrm{cm}^{2}

42\mathrm{cm}^{2}

Since this is a square, the base and height are the same, $7cm.$

\begin{aligned} \text{Area }&=\text{ base } \times \text{ height}\\\\ &=7 \times 7\\\\ &=49\mathrm{cm}^{2} \end{aligned}

55\mathrm{mm}^{2}

18\mathrm{mm}^{2}

36\mathrm{mm}^{2}

77\mathrm{mm}^{2}

The base is $11mm$ and the height is $5mm.$

\begin{aligned} \text{Area }&=\text{ base } \times \text{ height }\\\\ &=11 \times 5\\\\ &=55\mathrm{mm}^{2} \end{aligned}

260\mathrm{m}^{2}

33\mathrm{m}^{2}

16.5\mathrm{m}^{2}

130\mathrm{m}^{2}

\begin{aligned} \text{Area }&=\frac{1}{2} \times \text{ width } \times \text{ height}\\\\ &=\frac{1}{2} \times 20 \times 13\\\\ &=130\mathrm{m}^{2} \end{aligned}

36\mathrm{cm}^{2}

60\mathrm{cm}^{2}

26\mathrm{cm}^{2}

576\mathrm{cm}^{2}

\begin{aligned} \text{Area }&=\frac{1}{2}(a+b)h\\\\ &=\frac{1}{2} (8+12) \times 6\\\\ &=60\mathrm{cm}^{2} \end{aligned}

36\mathrm{mm}^{2}

13\mathrm{mm}^{2}

18\mathrm{mm}^{2}

72\mathrm{mm}^{2}

\begin{aligned} \text{Area }&=\frac{1}{2} \times \text{ base } \times \text{ height}\\\\ &=\frac{1}{2} \times 9 \times 4\\\\ &=18\mathrm{mm}^{2} \end{aligned}

69.1\mathrm{m}^{2}

1520\mathrm{m}^{2}

34.6\mathrm{m}^{2}

380\mathrm{m}^{2}

\begin{aligned} \text{Area }&=\pi r^{2}\\\\ &=\pi \times 11^{2}\\\\ &=380.132711\\\\ &=380\mathrm{m}^{2} \end{aligned}

418\mathrm{cm}^{2}

550\mathrm{cm}^{2}

561\mathrm{cm}^{2}

286\mathrm{cm}^{2}

How to Work Out Area Practice Question 7 Image 2

Shape A:

\begin{aligned} \text{Area }&=\frac{1}{2} \times \text{ base } \times \text{ height}\\\\ &=\frac{1}{2} \times 22 \times 12\\\\ &=132\mathrm{cm}^{2} \end{aligned}

Shape B:

\begin{aligned} \text{Area }&=\text{ base } \times { height}\\\\ &=22 \times 13\\\\ &=286\mathrm{cm}^{2} \end{aligned}

Total area: $132+286=418cm^2$

How to work out area GCSE questions

1. Work out the area of this shape

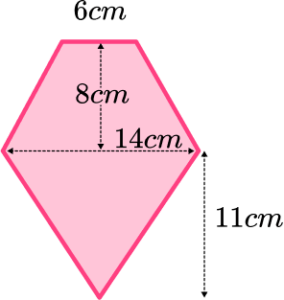
how to work out area gcse question 1 284x300

(3 marks)

Show answer

\text{Area of trapezium: }\frac{1}{2}(6+14) \times 8=80\mathrm{cm}^{2}

(1)

\text{Area of triangle: }\frac{1}{2} \times 14 \times 11 = 77\mathrm{cm}^{2}

(1)

Total area: $80 + 77 = 157cm^2$

(1)

2. Sean wants to paint both sides of a fence which is $20m$ long and $1.5m$ high.

One tin of the paint that Sean wants to use will cover $15m^2$ . How many tins of paint should Sean buy?

(3 marks)

Show answer

\text{Area of one side: } 20 \times 1.5 = 30\mathrm{m}^{2}

(1)

\text{Area of both sides: } 2 \times 30 = 60 \mathrm{m}^{2}

(1)

$60 \div 15 = 4$ tins

(1)

3. A shop sells pizza in two sizes: $10$ inches and $13$ inches. Would Louise get more pizza if she bought one large pizza or two small pizzas? Show how you decide.

How to Work Out Area GCSE Question 3

(3 marks)

Show answer

\text{Small pizza: Area }=\pi \times 5^{2} = 78.54\text{ square inches}

\text{Two small pizzas: } 2\times 78.54=157.08 \text{ square inches}

(1)

\text{Large pizza: Area} = \pi \times 6.5^{2} = 132.72 \text{ square inches}

(1)

Two small pizzas

(1)

Learning checklist

You have now learned how to:

Work out the area of triangles, quadrilaterals and circles

Work out the area of compound shapes

The next lessons are

Still stuck?

Prepare your KS4 students for maths GCSEs success with Third Space Learning. Weekly online one to one GCSE maths revision lessons delivered by expert maths tutors.