Solving Equations - Math Steps, Examples & Questions

High Impact Tutoring Built By Math Experts

Personalized standards-aligned one-on-one math tutoring for schools and districts

Request a demo

In order to access this I need to be confident with:

Algebraic expressions Combining like terms Expanding expressions Polynomials

Math resources Algebra Math equations

Solving equations

Here you will learn about solving equations, including linear and quadratic algebraic equations, and how to solve them.

Students will first learn about solving equations in grade $8$ as a part of expressions and equations, and again in high school as a part of reasoning with equations and inequalities.

Every week, we teach lessons on solving equations to students in schools and districts across the US as part of our online one-on-one math tutoring programs. On this page we’ve broken down everything we’ve learnt about teaching this topic effectively.

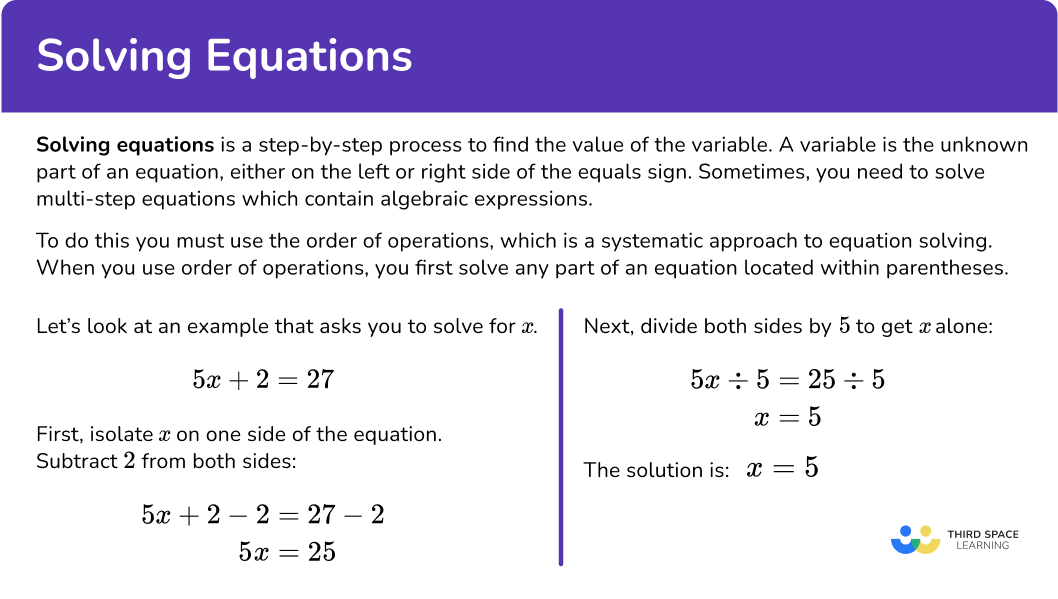
What is solving an equation?

Solving equations is a step-by-step process to find the value of the variable. A variable is the unknown part of an equation, either on the left or right side of the equals sign. Sometimes, you need to solve multi-step equations which contain algebraic expressions.

To do this, you must use the order of operations, which is a systematic approach to equation solving. When you use the order of operations, you first solve any part of an equation located within parentheses. An equation is a mathematical expression that contains an equals sign.

For example,

$\begin{aligned}y+6&=11\\\\ 3(x-3)&=12\\\\ \cfrac{2x+2}{4}&=\cfrac{x-3}{3}\\\\ 2x^{2}+3&x-2=0\end{aligned}$

There are two sides to an equation, with the left side being equal to the right side. Equations will often involve algebra and contain unknowns, or variables, which you often represent with letters such as $x$ or $y.$

You can solve simple equations and more complicated equations to work out the value of these unknowns. They could involve fractions, decimals or integers.

What is solving an equation?

Common Core State Standards

How does this relate to $8$ th grade and high school math?

Grade 8 – Expressions and Equations (8.EE.C.7)
Solve linear equations in one variable.

High school – Reasoning with Equations and Inequalities (HSA.REI.B.3)
Solve linear equations and inequalities in one variable, including equations with coefficients represented by letters.

[FREE] Solving Equations Worksheet (Grade 6 to 8)

Use this worksheet to check your grade 6 to 8 students’ understanding of solving equations. 15 questions with answers to identify areas of strength and support!

DOWNLOAD FREE

[FREE] Solving Equations Worksheet (Grade 6 to 8)

Use this worksheet to check your grade 6 to 8 students’ understanding of solving equations. 15 questions with answers to identify areas of strength and support!

DOWNLOAD FREE

How to solve equations

In order to solve equations, you need to work out the value of the unknown variable by adding, subtracting, multiplying or dividing both sides of the equation by the same value.

Combine like terms.
Simplify the equation by using the opposite operation to both sides.
Isolate the variable on one side of the equation.

Solving equations examples

Example 1: solve equations involving like terms

Solve for $x.$

5q-4q=9

Combine like terms.

Combine the $q$ terms on the left side of the equation. To do this, subtract $4q$ from both sides.

(5 q-4 q)=9-4 q

The goal is to simplify the equation by combining like terms. Subtracting $4q$ from both sides helps achieve this.

After you combine like terms, you are left with $q=9-4q.$

2Simplify the equation by using the opposite operation on both sides.

Add $4q$ to both sides to isolate $q$ to one side of the equation.

q+4q=9-4q+4q

The objective is to have all the $q$ terms on one side. Adding $4q$ to both sides accomplishes this.

After you move the variable to one side of the equation, you are left with $5q=9.$

3Isolate the variable on one side of the equation.

Divide both sides of the equation by $5$ to solve for $q.$

5q \div 5 = 9 \div 5

Dividing by $5$ allows you to isolate $q$ to one side of the equation in order to find the solution. After dividing both sides of the equation by $5,$ you are left with $q=1 \cfrac{4}{5} \, .$

Example 2: solve equations with variables on both sides

Solve for $x.$

7v=8–8v

Combine like terms.

Show step

Combine the $v$ terms on the same side of the equation. To do this, add $8v$ to both sides.

$7v+8v=8-8v+8v$

After combining like terms, you are left with the equation $15v=8.$

Simplify the equation by using the opposite operation on both sides and isolate the variable to one side.

Show step

Divide both sides of the equation by $15$ to solve for $v.$ This step will isolate $v$ to one side of the equation and allow you to solve.

$15v \div 15=8 \div 15$

The final solution to the equation $7v=8-8v$ is $\cfrac{8}{15} \, .$

Example 3: solve equations with the distributive property

Solve for $x.$

3(c-5)-4=2

Combine like terms by using the distributive property.

Show step

The $3$ outside the parentheses needs to be multiplied by both terms inside the parentheses. This is called the distributive property.

$\begin{aligned}& 3 \times c=3 c \\\\ & 3 \times(-5)=-15 \\\\ &3 c-15-4=2\end{aligned}$

Once the $3$ is distributed on the left side, rewrite the equation and combine like terms. In this case, the like terms are the constants on the left, $–15$ and $–4.$ Subtract $–4$ from $–15$ to get $–19.$

$3c-19=2$

Simplify the equation by using the opposite operation on both sides.

Show step

The goal is to isolate the variable, $c,$ on one side of the equation. By adding $19$ to both sides, you move the constant term to the other side.

$\begin{aligned}& 3 c-19+19=2+19 \\\\ & 3 c=21\end{aligned}$

Isolate the variable to one side of the equation.

Show step

To solve for $c,$ you want to get $c$ by itself.

Dividing both sides by $3$ accomplishes this.

On the left side, $\cfrac{3c}{3}$ simplifies to $c,$ and on the right, $\cfrac{21}{3}$ simplifies to $7.$

The final solution is $c=7.$

As an additional step, you can plug $7$ back into the original equation to check your work.

Example 4: solve linear equations

Solve for $x.$

2x+5=15

Combine like terms by simplifying.

Show step

Using steps to solve, you know that the goal is to isolate $x$ to one side of the equation. In order to do this, you must begin by subtracting from both sides of the equation.

$\begin{aligned} & 2x+5=15 \\\\ & 2x+5-5=15-5 \\\\ & 2x=10 \end{aligned}$

Continue to simplify the equation by using the opposite operation on both sides.

Show step

Continuing with steps to solve, you must divide both sides of the equation by $2$ to isolate $x$ to one side.

$\begin{aligned} & 2x \div 2=10 \div 2 \\\\ & x= 5 \end{aligned}$

Isolate the variable to one side of the equation and check your work.

Show step

Plugging in $5$ for $x$ in the original equation and making sure both sides are equal is an easy way to check your work. If the equation is not equal, you must check your steps.

$\begin{aligned}& 2(5)+5=15 \\\\ & 10+5=15 \\\\ & 15=15\end{aligned}$

Example 5: solve equations by factoring

Solve the following equation by factoring.

2x^2+3x-20=0

Combine like terms by factoring the equation by grouping.

Show step

Multiply the coefficient of the quadratic term by the constant term.

$2$ x $(-20) = -40$

Look for two numbers that multiply to give you $–40$ and add up to the coefficient of $3.$ In this case, the numbers are $8$ and $–5$ because $8$ x $-5=–40,$ and $8+–5=3.$

Split the middle term using those two numbers, $8$ and $–5.$ Rewrite the middle term using the numbers $8$ and $–5.$

$2x^2+8x-5x-20=0$

Group the terms in pairs and factor out the common factors.

$2x^2+8x-5x-20=2x(x + 4)-5(x+4)=0$

Now, you’ve factored the equation and are left with the following simpler equations $2x-5$ and $x+4.$

Simplify the equation by using the opposite operation on both sides.

Show step

This step relies on understanding the zero product property, which states that if two numbers multiply to give zero, then at least one of those numbers must equal zero.

Let’s relate this back to the factored equation $(2x-5)(x+4)=0$

Because of this property, either $(2x-5)=0$ or $(x+4)=0$

Isolate the variable for each equation and solve.

Show step

When solving these simpler equations, remember that you must apply each step to both sides of the equation to maintain balance.

$\begin{aligned}& 2 x-5=0 \\\\ & 2 x-5+5=0+5 \\\\ & 2 x=5 \\\\ & 2 x \div 2=5 \div 2 \\\\ & x=\cfrac{5}{2} \end{aligned}$

$\begin{aligned}& x+4=0 \\\\ & x+4-4=0-4 \\\\ & x=-4\end{aligned}$

The solution to this equation is $x=\cfrac{5}{2}$ and $x=-4.$

Example 6: solve quadratic equations

Solve the following quadratic equation.

x^{2}+2x-5=0

Combine like terms by factoring the quadratic equation when terms are isolated to one side.

Show step

To factorize a quadratic expression like this, you need to find two numbers that multiply to give $-5$ (the constant term) and add to give $+2$ (the coefficient of the $x$ term).

The two numbers that satisfy this are $-1$ and $+5.$

So you can split the middle term $2x$ into $-1x+5x: x^2-1x+5x-5-1x+5x$

Now you can take out common factors $x(x-1)+5(x-1).$

And since you have a common factor of $(x-1),$ you can simplify to $(x+5)(x-1).$

The numbers $-1$ and $5$ allow you to split the middle term into two terms that give you common factors, allowing you to simplify into the form $(x+5)(x-1).$

Simplify the equation by using the opposite operation on both sides.

Show step

This step relies on understanding the zero product property, which states that if two numbers multiply to give zero, then at least one of those numbers must equal zero.

Let’s relate this back to the factored equation $(x+5)(x-1)=0.$

Because of this property, either $(x+5)=0$ or $(x-1)=0.$

Isolate the variable for each equation and solve.

Show step

Now, you can solve the simple equations resulting from the zero product property.

$\begin{aligned}& x+5=0 \\\\ & x+5-5=0-5 \\\\ & x=-5 \\\\\\ & x-1=0 \\\\ & x-1+1=0+1 \\\\ & x=1\end{aligned}$

The solutions to this quadratic equation are $x=1$ and $x=-5.$

Teaching tips for solving equations

Use physical manipulatives like balance scales as a visual aid. Show how you need to keep both sides of the equation balanced, like a scale. Add or subtract the same thing from both sides to keep it balanced when solving. Use this method to practice various types of equations.

Emphasize the importance of undoing steps to isolate the variable. If you are solving for $x$ and $3$ is added to $x,$ subtracting $3$ undoes that step and isolates the variable $x.$

Relate equations to real-world, relevant examples for students. For example, word problems about tickets for sports games, cell phone plans, pizza parties, etc. can make the concepts click better.

Allow time for peer teaching and collaborative problem solving. Having students explain concepts to each other, work through examples on whiteboards, etc. reinforces the process and allows peers to ask clarifying questions. This type of scaffolding would be beneficial for all students, especially English-Language Learners. Provide supervision and feedback during the peer interactions.

Easy mistakes to make

Forgetting to distribute or combine like terms
One common mistake is neglecting to distribute a number across parentheses or combine like terms before isolating the variable. This error can lead to an incorrect simplified form of the equation.

Misapplying the distributive property
Incorrectly distributing a number across terms inside parentheses can result in errors. Students may forget to multiply each term within the parentheses by the distributing number, leading to an inaccurate equation.

Failing to perform the same operation on both sides
It’s crucial to perform the same operation on both sides of the equation to maintain balance. Forgetting this can result in an imbalanced equation and incorrect solutions.

Making calculation errors
Simple arithmetic mistakes, such as addition, subtraction, multiplication, or division errors, can occur during the solution process. Checking calculations is essential to avoid errors that may propagate through the steps.

Ignoring fractions or misapplying operations
When fractions are involved, students may forget to multiply or divide by the common denominator to eliminate them. Misapplying operations on fractions can lead to incorrect solutions or complications in the final answer.

Math equations
Rearranging equations
How to find the equation of a line
Solve equations with fractions
Linear equations
Writing linear equations
Substitution
Identity math
One step equation

Practice solving equations questions

x=3

x=4

x=14

x=3.5

4x-2=14

Add $2$ to both sides.

4x=16

Divide both sides by $4.$

x=4

x=7

x=4

x=8

x=6

3x-8=x+6

Add $8$ to both sides.

3x=x+14

Subtract $x$ from both sides.

2x=14

Divide both sides by $2.$

x=7

x=2

x=–3

x=–13

x=13

3(x+3)=2(x-2)

Expanding the parentheses.

3x+9=2x-4

Subtract $9$ from both sides.

3x=2x-13

Subtract $2x$ from both sides.

x=-13

x=2

x=–3

x=–13

x=13

\cfrac{2 x+2}{3}=\cfrac{x-3}{2}

Multiply by $6$ (the lowest common denominator) and simplify.

2(2x+2)=3(x-3)

Expand the parentheses.

4x+4=3x-9

Subtract $4$ from both sides.

4x=3x-13

Subtract $3x$ from both sides.

x=-13

x=±4

x=±2

x=±3

x=±6

\cfrac{3 x^{2}}{2}=24

Multiply both sides by $2.$

3 x^{2}=48

Divide both sides by $3.$

x^{2}=16

Square root both sides.

x=\pm 4

x=-3, \; x=10

x=-3, \; x=-10

x=3, \; x=10

x=3, \; x=-10

x^{2}-13 x+30=0

Use factoring to find simpler equations.

(x-3)(x-10)=0

Set each set of parentheses equal to zero and solve.

$x=3$ or $x=10$

Solving equations FAQs

What is the first step in solving a simple linear equation?

The first step in solving a simple linear equation is to simplify both sides by combining like terms. This involves adding or subtracting terms to isolate the variable on one side of the equation.

Why is it important to perform the same operation on both sides of the equation when solving it?

Performing the same operation on both sides of the equation maintains the equality. This ensures that any change made to one side is also made to the other, keeping the equation balanced and preserving the solutions.

How do I handle variables on both sides of the equation when solving for a variable?

To handle variables on both sides of the equation, start by combining like terms on each side. Then, move all terms involving the variable to one side by adding or subtracting, and simplify to isolate the variable. Finally, perform any necessary operations to solve for the variable.

What should I do if there are fractions in the equation when solving for a variable?

To deal with fractions in an equation, aim to eliminate them by multiplying both sides of the equation by the least common denominator. This helps simplify the equation and make it easier to isolate the variable. Afterward, proceed with the regular steps of solving the equation.

The next lessons are

Still stuck?

At Third Space Learning, we specialize in helping teachers and school leaders to provide personalized math support for more of their students through high-quality, online one-on-one math tutoring delivered by subject experts.

Each week, our tutors support thousands of students who are at risk of not meeting their grade-level expectations, and help accelerate their progress and boost their confidence.